The calculation of the Madelung constant

A previous article showed that the electrostatic term of the lattice energy of a crystal contains a factor (A) that depends on the type of crystalline reticulum. This article will look more in detail how to calculate this term and its value for a simple ionic system.

The total Coulomb interaction energy of a crystal is given by the sum of the single pair interaction terms:

$\displaystyle V_{AB} = \frac{e^2}{4\pi\epsilon_0} \frac{Z_AZ_B}{r_{AB}} \hfill (1)$

for ions with charges q_A and q_B and distance r_AB. The sum extends to all pairs of ions present in the solid for any crystalline structure.

The sum converges very slowly because the first neighbours give a first substantial contribution with a negative summation term. Second neighbour atoms produce a slightly weaker positive term. It then continues to infinity with terms of alternating signs by smaller and smaller values. In this way, the resulting effect will be that the attraction between cations and anions predominates and provides a favourable negative contribution to the solid’s energy.

Infinite one-dimensional reticulum.

In a mono-dimensional reticulum with alternate cations and anions at intervals of constant of length d, and having charges q_A=+Z and q_B=-Z, the interaction of one ion with all others is given by the series:

$V = -\frac{2Z^2}{d} + \frac{2Z^2}{2d} - \frac{2Z^2}{3d} + \frac{2Z^2}{4d} - ...$

$= -\frac{2Z^2}{d} \left(1 -\frac{1}{2} +\frac{1}{3} -\frac{1}{4} + ... \right)$

$= -\frac{2Z^2}{d} \ln 2$

(the factor 2 derives from the fact that each ion in the reticulum had on both sides identical ions). The sum depends only on the type of reticulum and by the distance d between the centres of adjacent particles:

$\displaystyle V = -\frac{e^2}{4\pi\epsilon_0} \frac{Z^2}{d} (2 \ln 2) \hfill (2)$

In the case of one-dimensional lattice, $\displaystyle V$ contain the term ${\frac{Z^2}{d}}$ depending on the charge type and reticulum. The factor 2ln 2 (= 1,3862944) is called Madelung (A) constant and characterizes the reticulum’s symmetry.

Infinite two-dimensional reticulum

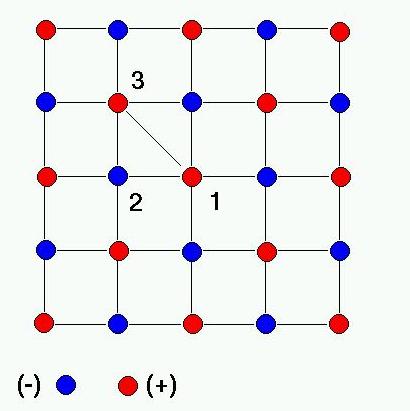
In the case of a two-dimensional reticulum, the cations and the anions are disposed at regular intervals on contiguous squares of length d and with ${q_A = +Z}$ e ${q_B = -Z}$ . Considering as centre the ion (1) in the above diagram and moving radially from it, the interaction of this ion with all the others is given by:

$V = -\frac{4Z^2}{d} + \frac{4Z^2}{\sqrt{2} d} - \frac{8Z^2}{\sqrt{5} d} + \frac{4Z^2}{2\sqrt{2} d} + \frac{4Z^2}{2 d} ...$

$= -\frac{4Z^2}{d} \left(1 -\frac{1}{\sqrt{2}} +\frac{2}{\sqrt{5}} -\frac{1}{2\sqrt{2}} + \frac{1}{2 } ... \right)$

Infinite three-dimensional reticulum

This formulation can be easily extended to a three-dimensional lattice.

In a simple solid, like the NaCl in the figure, the Madelung constant depends on the crystal type and by inter-ionic distances. The Madelung constant for a three-dimensional lattice is calculated by starting from an ion placed at the lattice’s centre and then moving radially until the first $n_1$ neighbours, having charges of opposite value, at distance $d_1$ are found. Then, by moving radially to the central ion, the second $n_2$ neighbours (having the same electric charges of the central one) are located at the distance $d_2$ . The procedure is then further extended in the same way for a group of neighbour atoms organized in a concentric shell around the atoms’ previous group. The Madelung constant, in this case, will be defined by the summation:

$\displaystyle A = \sum_i \left(-sgn(q_A q_B)\right) n_i \frac{1}{(d_i/d)} \hfill (3)$ ,

where ${-sgn(q_A q_B)}$ indicates the sign of each term of the sum, and it is positive if the ions have opposite electric charges (attraction) and negative in sign if they have an equal charge (repulsion), and ${r^+}$ + ${r^-}$ is the sum of ionic radii of the ions in the lattice.

Example

As an example, we consider the Na+ in NaCl. It has 6 first neighbour’s Cl^– (n₁ = 6 at a distance) d₁=d; then there are 12 second Na⁺ as second neighbours (n₂=12) at a distance ; 8 third neighbours Cl^– (n₃=8) at a distance ; etc. Therefore, the resulting series is given by the following expression:

$\displaystyle A = + 6 - \frac{12}{\sqrt 2} + \frac{8}{\sqrt 3} - ... \hfill (4)$

The potential energy for one mole in the crystalline structure is given by:

$\displaystyle V = - A N_A \frac{e^2}{4\pi\epsilon_0} \frac{Z_AZ_B}{d} \hfill(5)$

TiO_2 — **Table 1: Constants of Madelung for some solids**

Al_2O_3 — **Table 1: Constants of Madelung for some solids**

^* This this the value of A divided by the number n of ions in the chemical formula of the salt.

As shown for the NaCl (6,6) and CsCl (8,8) structures, the constant of Madelung increases with the coordination number as the most important contribution comes from the first neighbours.

If you have found interesting and useful my article, do not forget to press “Like it” and subscribe for updates on new ones!

About Danilo Roccatano

I have a Doctorate in chemistry at the University of Roma “La Sapienza”. I led educational and research activities at different universities in Italy, The Netherlands, Germany and now in the UK. I am fascinated by the study of nature with theoretical models and computational. For years, my scientific research is focused on the study of molecular systems of biological interest using the technique of Molecular Dynamics simulation. I have developed a server (the link is in one of my post) for statistical analysis at the amino acid level of the effect of random mutations induced by random mutagenesis methods. I am also very active in the didactic activity in physical chemistry, computational chemistry, and molecular modeling. I have several other interests and hobbies as video/photography, robotics, computer vision, electronics, programming, microscopy, entomology, recreational mathematics and computational linguistics.

View all posts by Danilo Roccatano →

This entry was posted in Science Topics. Bookmark the permalink.

1 Response to The calculation of the Madelung constant

John Veranth says:

January 26, 2019 at 2:06 am

Very nice presentation to introduce someone to the topic. Well written, concise, and emphasizing both the fundamentals and numerical values. Compared to the “hair grind” summations on Wolfram or the precise but unclear discussion on Wikipedia this posting is where I would recommend a student start reading.

LikeLiked by 2 people

Structure	A	A/n*	Coordination
CsCl	1.763	0.88	(8,8)
Halite (NaCl)	1.748	0.87	(6,6)
Fluorite (CaF₂)	2.519	0.84	(8,4)
Wurtzite (ZnS)	1.641	0.82	(4,4)
CdCl₂	2.244	0.75	(6,3)
CdI₂	2.191	0.73	(6,3)
Rutile ( $TiO_2$ )	2.408	0.80	(6,3)
Curundum ( $Al_2O_3$ )	4.172	0.83	(6,4)

M	T	W	T	F	S	S
						1
2	3	4	5	6	7	8
9	10	11	12	13	14	15
16	17	18	19	20	21	22
23	24	25	26	27	28	29
30	31